Jason A. Burdette
ESM 4984
Farkas2
Vacancies and Free Surfaces in Fe
This assignment involved the analysis of two different crystal structures of Iron. Iron is of great importance to engineering applications and perhaps its most obvious and common application is its combination with carbon to produce steel. Although its heyday has certainly passed, it could be argued that steel is one of the most important engineering materials of all time for its use in such applications as structures, tools, weapons, automobiles and many others. Although iron is most commonly found in its BCC (body-centered cubic) lattice structure, it can also exist as FCC (face-centered cubic). Both types of crystals were simulated in this analysis. Physical parameters for both types of crystal lattice structure are shown below:
FCC: lattice parameter, a = 3.515 A
cohesive energy, Ec = 4.196 eV
BCC: lattice parameter, a = 2.87 A
cohesive energy, Ec = 4.28 eV
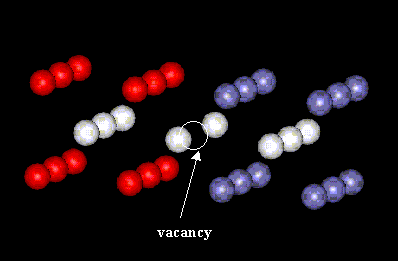
The first part of the assignment involved the removal of an atom from both the FCC and BCC structures. Figure 1 shows the section of the block containing the defect for FCC model and Figure 2 is for the BCC structure.

Figure 1: FCC unit cells containing vacancy
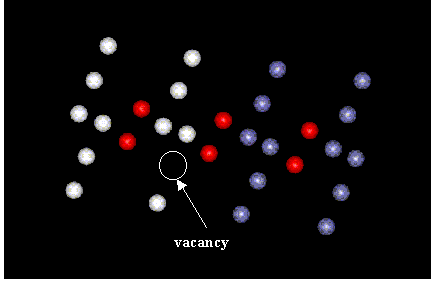
Figure 2: BCC unit cells containing vacancy
These two regions were analyzed both for the vacancy formation energy (the energy required to remove the atom) and for the atomic displacements of the neighboring atoms. The atomic displacements were found by monitoring the distance between atoms and comparing the values with the original lattice parameter values. The defect energy, Ed, was calculated and used to obtain the vacancy formation energy.
For FCC, note that the measured distances are compared with the length of two lattice parameters so the original distance is 2a = 7.03 A. At the vacancy, the distance is 7.08 A. There is less attraction between the atoms than if the vacancy was not present. The neighboring atoms are spaced by 7.02 A, which is less than the original value for 2A. Although only a small section of the block is shown, this trend of alternating positive and negative atomic displacements would continue, with the displacements getting gradually smaller farther away from the vacancy (meaning that the atoms closer to the vacancy are more affected by the vacancy than those farther away).
Similarly, for BCC, the edge closest to the vacancy was measured as 5.82 A, greater than 2a = 5.56 A. The next edge measured 5.68 A. The following edge, 5.74 A. In this case, note that the displacements alternated from greater to smaller, but didnít alternate from positive to negative, as I expected. Again, only a small section of the block was analyzed. Perhaps if a greater portion of the block had been included, negative displacements would have been observed.
The defect energy, Ed, was calculated and used to obtain the vacancy formation energy, Evf, or the amount of energy required to remove the atom, by the calculation:
Evf = Ed - Ec
FCC: vacancy formation energy = 1.66 eV
BCC: vacancy formation energy = 1.71 eV
Thus, the BCC block requires a greater amount of energy than the FCC to remove an atom and create a vacancy.
The next part of the assignment involved the creation of a planar defect in a block of BCC iron. Figure 3 shows the section of the block containing the defect.
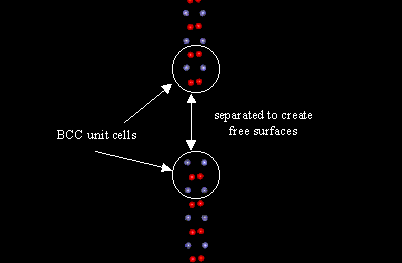
Figure 3: BCC unit cells separated to create free surface
Again, the atomic displacements were observed. This time, however, the free surface energy (the energy required to separate the two sections) was calculated.
The displacements for the neighboring atoms in this case seemed to follow a peculiar trend. Rather than alternating from large to small or positive to negative as the atoms did in the presence of a vacancy, they alternated in the repeating pattern of two large displacements, followed by two small displacements. This was contrary to what I expected and I offer no guess as to why this occurred.
The surface energy, or more precisely, the specific surface energy for one plane, is calculated by:
Esp (plane) = E/[{(tx*dx)/ix}*{(tz*dz)/iz}]*16/2
where: E = energy
tx, tz = buffer thickness in x and z directions
dx, dz = dimensions of inner block in x and z directions
ix, iz = inner block in x and z directions
16 = conversion to eV
2 = division of 2 surfaces to yield energy for 1 surface only
specific surface energy = 0.381 eV/A2
In summary, the BCC crystal structure required more energy than the FCC structure to create a vacancy. The effect of this vacancy on the surrounding atoms was slightly different depending on the lattice structure. In BCC, the displacements alternated from large to small, gradually decaying to zero, but remained positive, at least in the region analyzed. The FCC displacements also decayed to zero, but alternated from positive to negative. The planar defect in the BCC block was analyzed for its specific surface energy, but no simulation was run with FCC for comparison.