Jason A

Jason A. Burdette

ESM 4984

Farkas3

Dislocation Emission in Fe

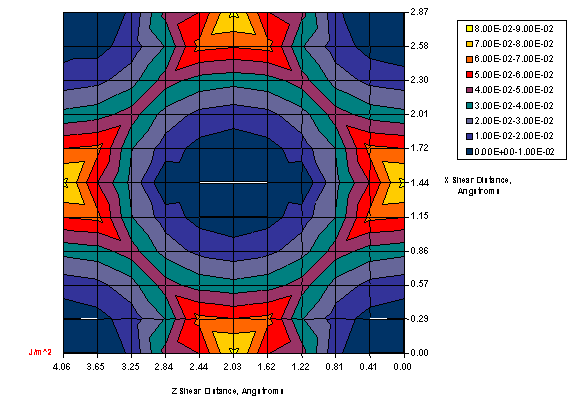
The purpose of this assignment was to simulate shear planar faults in BCC (base-centered cubic) Iron. The analysis was performed on the y=0 shear plane by specifying shear vectors in the x and z directions. That is, the plane was shifted incrementally from 0 to a = 2.87 A in the x-direction and from 0 to aÖ 2 = 4.06 A in the z-direction. The energy of the shear fault was calculated at each position and the value recorded in a spreadsheet. The values were plotted as contours in the y=0 plane to show regions of high and low energy. Figure 1 shows this contour plot.

Figure 1: Contour plot of obtained energies of planar faults as a function of x and z shear distance

Note that the energy is lowest at the corners and in the center of this section of the y=0 plane. This makes sense as atoms are located at these locations. The atoms tend to their lowest energy states and a high amount of energy needs to be put into the system in order to move them. The brightly colored regions between atom locations represent high energies. It is very difficult to shear this plane such that the atoms move to these high energy regions.

To visualize this, consider that the atoms, in their relaxed state, rest in the vacancies between atoms on the layer below them. This is low energy configuration is the natural orientation of the atoms. If a shear fault was introduced such that the atoms were positioned directly above the atoms in the layer below, they would tend to fall back from this high energy state into their lower energy state. Also note from this plot that if the plane is shifted so that a particular atom is past the high energy region, it will tend towards the next successive low energy state, (the original position of its neighboring atom).

This type of study is of vital importance in the field of ductility of metals. These contours basically serve as maps of atomic locations (indicated by low energy regions). The ductility of a metal would depend strongly on the amount of energy needed to dislocate the atom (move it to its next low energy state). Higher energy between atom locations would suggest that a great deal of strain energy needs to be put into the material to yield this dislocation. Lower variations in energy would suggest a more ductile material.