Defect Energies in Iron

a study of BCC and FCC iron

To learn about iron you may try some of the following links:

http://www.shef.ac.uk/chemistry/web-elements/nofr-index/Fe.html
http://www.stlewis.publib.nf.ca/minerals/iron.htm
http://home1.pacific.net.sg/~mingly/mnglyfaq.htm
http://www.chem.ualberta.ca/courses/plambeck/p102/p02263.htm
http://me.mit.edu/2.01/Taxonomy/Characteristics/Iron.html
http://www.healthworld.com/othersites/lilipoh/iron.htm
(found with www.excite.com searching +iron +properties +metal)

Here are some of the highlights.

DESCRIPTION

Iron, a silvery white solid metal, appears in Group VIII of the periodic table as a transition element. Its atomic number is 26, and its atomic weight is 55.847. Iron is notable among the elements in the abundance of its ores and the vast number of useful alloys that can be formulated with iron as the major constituent. Iron is also biologically important.

In its pure form, iron is rather soft and is malleable and ductile at room temperature. It melts at 1,535 deg C and boils at 3,000 deg C. Pure iron can exist in two structural types, or allotropic forms. At room temperature the iron atoms are arranged in a body-centered cubic lattice called the a-form, which is transformed at 910 deg C into a cubic close-packed structure called the gamma-form. At 1,390 deg C iron returns to a body-centered cubic structure, called the delta-form.

APPLICATION

Iron is abundant and easily obtainable from its ores. Its desirable mechanical and magnetic properties, as well as its resistance to corrosion, may be improved by mixing iron with other elements, frequently metals, to form alloys.

Perhaps the most important alloy of iron is steel, which contains up to approximately 2% carbon. Steels that contain about 0.25% carbon are called mild steels; those with about 0.45% carbon are medium steels; and those with 0.60% to 2% carbon are high-carbon steels. Within this range, the greater the carbon content, the greater the tensile strength of the steel. The hardness of steel may be substantially increased by heating the metal until it is red hot and then quickly cooling it, a process known as quench hardening. An important component of many steels is cementite, a carbon-iron compound. Mild steels are ductile and are fabricated into sheets, wire, or pipe; the harder medium steels are used to make structural steel. High-carbon steels, which are extremely hard and brittle, are used in tools and cutting instruments.

The addition of other materials in alloys (for example, manganese or silicon) also increases the hardness of steel. The inclusion of tungsten permits high-speed drills and cutting tools to remain hard even when used at high temperatures. The inclusion of chromium and nickel improves the corrosion resistance of the steel and, within certain limits of composition, is called stainless steel. A common stainless steel contains 0.15% carbon, 18% chromium, and 8% nickel and is used in cooking utensils and food-processing equipment. The inclusion of silicon, ranging from 1 to 5%, results in an alloy that is hard and highly magnetic. An alloy with cobalt is used for permanent magnets.

THE SIMULATION

Computer simulations of defect energies were run for both vacancies in BCC and FCC iron as well as free surface energy for BCC iron.

Parameters for the simulations included the following facts about iron:

FCC: lattice parameter = 3.515Å, cohesive energy = 4.196eV

BCC: lattice parameter = 2.87Å, cohesive energy = 4.28eV

The output files for the FCC vacancy, the BCC vacancy, and the BCC free surface energy are just one click away.

And the xyz files for the FCC vacancy, the BCC vacancy, and the BCC free surface energy are also just one click away.

THE RESULTS


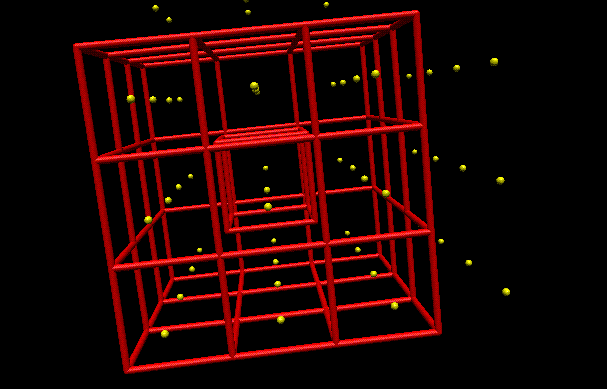
Figure 1 : Vacancy in a BCC crystal lattice.

For visualization purposes, the lattice atoms (arbitrarily designated so) are red and connected while the body centered atoms are yellow. The vacancy is "located" within the inner red cube.

The BCC iron vacancy simulation resulted in a defect energy of 5.988eV.


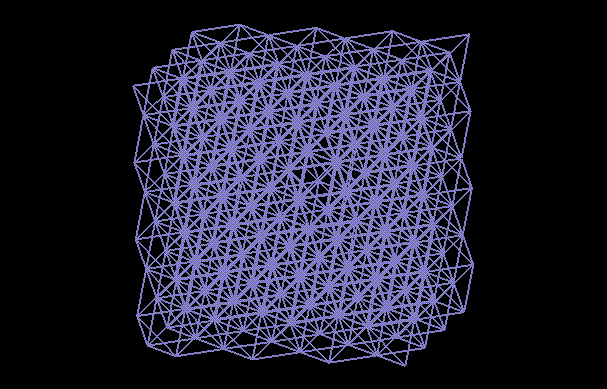
Figure 2 : Vacancy in a FCC crystal lattice.


Figure 3 : Vacancy in a FCC crystal lattice.


Figure 4 : Vacancy in a FCC crystal lattice.

The above three figures show the simulation of a defect in FCC iron. Figure 2 is included for the benefit of the reader. It is not "spiffed up," but rather shows the size and complexity of the simulation. The vacancy is the irregularity toward the center of the figure. For visualization purposes, both Figure 3 (ortho projection) and Figure 4 (perspective projection) show diagonals of all lattice faces. These are the "nearest neighbor" bonds. The lattice face that "contains" the vacancy (a "face" atom) is emphasized by the yellow color of its face's corners.

The FCC iron vacancy simulation resulted in a defect energy of 5.857eV.


Figure 5 : Free surface in a BCC crystal lattice.

The above figure looks rather odd, but that is because of the computational saving calculation scheme of the simulation software. First of all, the BCC lattice can be defined by only two atoms and three perpendicular vectors. Secondly, the symmetry of the surface in the x and z-directions means that periodic boundary conditions can be utilized to make computation faster. Therefore, all that you see is one lattice atom and one body centered atom per cubic unit. The surfaces have been seperated by 20Å so that they no longer interact and the change in energy can be computed as the free surface energy.

The following key data was extracted from bcc_sur.out for calculation of the defect (free surface) energy. 

   OUTER  BLOCK IS    7  BY  33  BY   7
   BUFFER THICKNESS   3  BY   3  BY   3
   INNER  BLOCK IS    1  BY  20  BY   1
   DIMENSIONS OF THE INNER BLOCK:   2.87000  57.40000   2.87000

   CYCLIC BOUNDARY IN X DIRECTION
   CYCLIC BOUNDARY IN Z DIRECTION

   THE PERFECT LATTICE BLOCK CONTAINS:
   TOTAL NUMBER OF  ATOMS:  NTOT  =     66
   NUMBER OF  FREE  ATOMS:  NFREE =     40
   NUMBER OF BUFFER ATOMS:              12
   NUMBER OF FIXED  ATOMS:              14

   MAXIMUM ATOMIC DISPLACEMENT DUE TO RELAXATION: 0.13848
   MAXIMUM FORCE AFTER RELAXATION: 0.000361
 
   ----------------------------------------------------
   MINIMUM ENERGY OF THE BLOCK: -0.1893485D+03
   SPECIFIC ENERGY OF THE PLANAR DEFECT:  0.4032043D+01
   ----------------------------------------------------

This calculation to correct for a software glitch was a little involved, but it went like this: 

40+12=52atoms   
     *4.28eV=222.56eV   
            -189.35=33.21eV   
                   /(3/1*2.87)^2=0.448eV/Angstrom
                                *16=7.167J/m^2
                                   /2=3.58J/m^2 per surface

The BCC free surface simulation in the (1 0 0) plane resulted in a defect energy of 0.448eV/Å ² or 3.58J/m ².