

Next: Deformation Field in an
Up: No Title
Previous: Principle of Minimum Potential
The solution of the boundary value problem
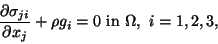 |
(10.1) |
 |
(10.2) |
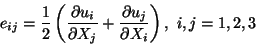 |
(10.3) |
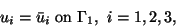 |
(10.4) |
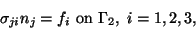 |
(10.5) |
is unique provided that
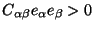 for every
for every
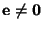 , and
, and 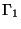 is not empty. If
is not empty. If 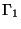 is
empty, then the solution is unique only to within a rigid body motion. For an
isotropic linear elastic material, the condition
is
empty, then the solution is unique only to within a rigid body motion. For an
isotropic linear elastic material, the condition
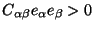 is equivalent to
is equivalent to
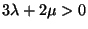 and
and 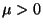 .
.
A consequence of this uniqueness theorem is that if a solution of equations
(10.1)-(10.5) has been found by any means, then it is the only solution of the
problem.


Next: Deformation Field in an
Up: No Title
Previous: Principle of Minimum Potential
Norma Guynn
1998-09-09